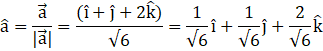Find the unit vector in the direction of the vector ![]()
We know that unit vector means that the magnitude of the vector is 1(unit).
It is defined as ![]()
So, we find the magnitude of ![]() first.
first.
![]()
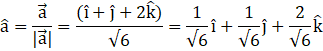
13
Find the unit vector in the direction of the vector ![]()
We know that unit vector means that the magnitude of the vector is 1(unit).
It is defined as ![]()
So, we find the magnitude of ![]() first.
first.
![]()