The principle of ‘parallax’ in section 2.3.1 is used in the determination of distances of very distant stars. The baseline AB is the line joining the Earth’s two locations six months apart in its orbit around the Sun. That is, the baseline is about the diameter of the Earth’s orbit � 3 × 1011m. However, even the nearest stars are so distant that with such a long baseline, they show parallax only of the order of 1” (second) of arc or so. A parsec is a convenient unit of length on the astronomical scale. It is the distance of an object that will show a parallax of 1” (second) of arc from opposite ends of a baseline equal to the distance from the Earth to the Sun. How much is a parsec in terms of metres?
Given,
Diameter of Earth’s orbit, d = 3 × 1011 m
Radius of earth’s orbit, r = d/2
⇒ r = (3×1011m)/2 = 1.5×1011 m
Parallax angle, θ = 1”
⇒ θ = 1’/60
⇒ θ = 1°/(60×60)
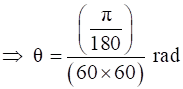

We know,
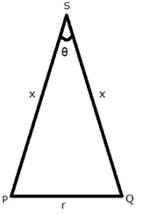
θ = Arc/Radius
⇒ θ = r/x
⇒ x = r/θ
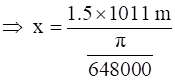
⇒ x = 3.09 × 1016 m
Hence, 1 parsec = 3.09 × 1016 m
NOTE: The parsec is a unit of length used to measure large distances to astronomical objects outside the Solar System. Parallax is a difference in the apparent position of an object viewed along two different lines of sight, and is measured by the angle or semi-angle of inclination between those two lines of sight.