A man walking briskly in rain with speed v must slant his umbrella forward making an angle θ with the vertical. A student derives the following relation between θ and v: tan θ = v and checks that the relation has a correct limit: as v → 0 , θ → 0, as expected. (We are assuming there is no strong wind and that the rain falls vertically for a stationary man). Do you think this relation can be correct? If not, guess the correct relation.

Question

Philoid · Accepted Answer

Dimensions of tan θ = M⁰L⁰T⁰

(∵ All trigonometric functions have no units)

Dimensions for v (velocity) = M⁰L¹T^-1

∴ The equation, tan θ = v is Dimensionally incorrect.

To balance the equation,

Let the speed of the rainfall be V, then we can make R.H.S dimensionless by dividing with V.

tan θ =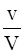

(∵ velocity dimension = M⁰L¹T^-1 always)

Now, the equation is dimensionally balanced and correct.