Two trains A and B of length 400 m each are moving on two parallel tracks with a uniform speed of 72 km/h in the same direction, with A ahead of B. The driver of B decides to overtake A and accelerates by 1 ms-2. If after 50 s, the guard of B just brushes past the driver of A, what was the original distance between them?
Given,
Initial velocity of both train A and train B, u = 72 km/h
=
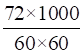= 20 m/s
Length of each train, l = 400 m
Acceleration of train A = 0 ms-2
Acceleration of train B = 1 ms-2
Time to overtake, t = 50 s
Distance to be covered to overtake,
Let, Distance travelled by train B in t, sB
Distance travelled by train A in t, sA
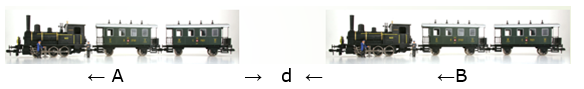
We have,
sB + sA= initial distance between trains (d) + (2×length of the train)
= d + (2×400) m
From 1st equation of motion,
v = u + at
where,
v = Final velocity
u = Initial velocity
a = Acceleration/Deceleration
t = Time
Final velocity of train A, vA = 20 + 0 = 20 m/s
Final velocity of train B, vB = 20 + (1×50) = 70 m/s
From 2nd equation of motion,
s = ut + 0.5at2
where,
u = Initial velocity
a = Acceleration/Deceleration
s = Distance covered
t = Time
∴ sA = (20×50) + (0.5×0×502) = 1000 m
sB = (20×50) + (0.5×1×502) = 2250 m
∴ Initial distance between trains, d = 2250 – (2×400)-1000
= 450 m