Explain clearly, with examples, the distinction between:
(a) Magnitude of displacement (sometimes called distance) over an interval of time, and the total length of path covered by a particle over the same interval;
(b) Magnitude of average velocity over an interval of time, and the average speed over the same interval. [Average speed of a particle over an interval of time is defined as the total path length divided by the time interval]. Show in both (a) and (b) that the second quantity is either greater than or equal to the first. When is the equality sign true? [For simplicity, consider one-dimensional motion only].
(a) The shortest path between two points in a travel is defined as Displacement and the actual path traveled is called Distance (or) Total length of the path in same interval.

In above figure, AB is Displacement and AC-CD-DB is distance or Total length of the path in the same time interval T.
(b) Average velocity, v =  m/s
m/s
Average speed, s =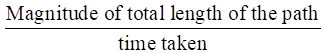 m/s
m/s
From the figure,
v =  m/s
m/s
s = 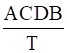 m/s
m/s