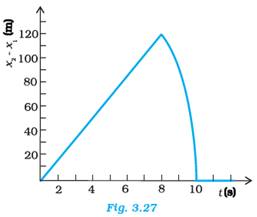
Two stones are thrown up simultaneously from the edge of a cliff 200 m high with initial speeds of 15 m/s and 30 m/s. Verify that the graph shown in Fig. 3.27 correctly represents the time variation of the relative position of the second stone with respect to the first. Neglect air resistance and assume that the stones do not rebound after hitting the ground. Take g = 10 m/s2. Give the equations for the linear and curved parts of the plot.
For first stone:
Initial velocity, u1 = 15 m/s
Acceleration, a = -g = -10 m/s2
Using the relation,
xf = x0 + ut + 0.5at2 ……………..(1)
Where,
xf = Final position = here x1, ground, 0 m
x0 = Initial position = here height of the cliff, 200 m
u = Initial velocity = u1
a = Acceleration
t = time
⇒ 0 = 200 + 5t + (0.5×10)t2
By solving, t = 8 s
For second stone:
Initial velocity, u2 = 30 m/s
Acceleration, a = -g = -10 m/s2
Using the relation,
xf = x0 + ut + 0.5at2 …………..(2)
Where,
xf = Final position = here x2, ground, 0 m
x0 = Initial position = here height of the cliff, 200 m
u = Initial velocity = u2
a = Acceleration
t = time
⇒ 0 = 200 + 30t + 5t2
By solving, t = 10 s
Subtracting equation 1 from equation 2 to we get,
x2-x1 = 15t ………….. (3)
Equation 3 represent straight line.
Due to this linear relation between (x2-x1) and t , the remains straight line till 8 s.
So, Maximum separation between two stones occurs at t = 8 s.
∴(x2-x1)max = 15×8 = 120 m
After 8 s, only second stone is in motion whose variation is given by the quadratic equation,
(x2-x1) = 200 + 30t + 5t2
Hence, the equations of linear and curved path are given by,
(x2-x1) = 15t (linear)
(x2-x1) = 200 + 30t + 5t2 (curved)