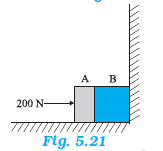
Two bodies A and B of masses 5 kg and 10 kg in contact with each other rest on a table against a rigid wall (Fig. 5.21). The coefficient of friction between the bodies and the table is 0.15. A force of 200 N is applied horizontally to A. What are (a) the reaction of the partition (b) the action reaction forces between A and B? What happens when the wall is removed? Does the answer to (b) change, when the bodies are in motion? Ignore the difference between μs and μk.
Given:
Mass of body A, MA = 5 Kg
Mass of body B, MB = 10Kg
Coefficient of friction between bodies and surface, � = 0.15
Force applied on block A, F = 200N
(A) The reaction force at partition, R
The force of 200 N is acting rightwards, a frictional force will act in leftward direction against the impending motion.
Using Newton’s second law,
F’ = F- fs
F’ = 200- ( �× (M �a + MB) g)
F’ = 200N- (0.15 × (10 + 5) Kg× 10ms-2)
F’ = 177.5 N
Net force acting in rightward direction at the partition is 177. 5 N.
We can conclude using 3rd law of motion that the normal force at the partition is 177.5 N in leftward direction.
(B) Force of friction on body A,
fA = �MAg
→ fA = 0.15× 5Kg × 10ms-2
→ fA = 7.5 N
This force acts leftwards.
Net force exerted by A on B ,
FNet = F- fA
FNet = 200N - 7.5N
FNet = 192.5 N
This force acts rightwards.
According to Newton’s 3rd law we can conclude that force by B on A is also 192.5 N in leftward direction.
If the Wall is removed, the bodies will start in the direction of applied force.
For motion of combined body,
From (A), we see that Net force on the bodies, F = 177.5 N
According to Newton’s second law, we can write,
F = (MA + MB)× a
a = F/ (MA + MB)
a = 177.5/ (5 + 10)
a = 11.83 ms-2
Net force on body A,
F = MA× a
F = 5Kg× 11.83 ms-2
F = 59.15 N
Net force on body, B by Body A,
F = 192.5- 59.15
F = 133.35 N
This force acts in the direction of motion of body. An equal amount of force is applied by body B on A, in opposite direction.