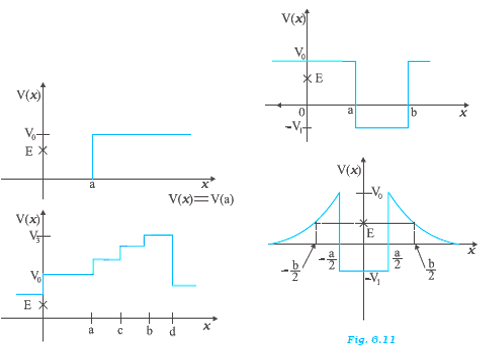
Given in Fig. 6.11 are examples of some potential energy functions in one dimension. The total energy of the particle is indicated by a cross on the ordinate axis. In each case, specify the regions, if any, in which the particle cannot be found for the given energy. Also, indicate the minimum total energy the particle must have in each case. Think of simple physical contexts for which these potential energy shapes are relevant.
The kinetic energy of a particle of mass m moving with a velocity v is given as
K=
Since m is always positive, K is also always positive.
The total energy (E) of a particle is equal to the sum of Kinetic energy (K) and Potential energy (PE).
⇒ E = K + PE
⇒ K = E – PE
a) For x>a, PE(=V0)>E.
This means that the kinetic energy is negative. Hence, the particle cannot exist in the region x>a.
b) For x<a and x>b, PE(=V0)>E.
This means that the kinetic energy is negative. Hence, the particle cannot exist in the region x<a and x>b.
c) In the region a<x<b, the kinetic energy is positive. The potential energy is -V1. So, K = E-(-V1) = E + V1.
In order for K to be positive, E> -V1. So, the minimum total energy of the particle is -V1.
d) For the regions -b/2<x<-a/2 and a/2<x<b/2, the potential energy of the particle is greater than the total energy which suggests that the kinetic energy is negative in this region. Hence, the particle cannot exist in this region.
The lowest potential energy is -V1. So, K = E-(-V1) = E+V1.
For K to be positive, E should be greater than -V1. So, minimum total energy is -V1.