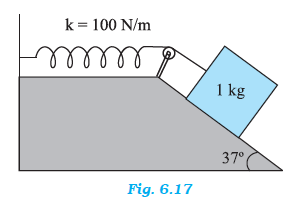
A 1 kg block situated on a rough incline is connected to a spring of spring constant 100 N m–1 as shown in Fig. 6.17. The block is released from rest with the spring in the unstretched position. The block moves 10 cm down the incline before coming to rest. Find the coefficient of friction between the block and the incline. Assume that the spring has a negligible mass and the pulley is frictionless.
Given,
Mass of the block, m = 1 kg
Spring constant, k = 100 N m-1
Displacement of the block, s = 10 cm = 0.1 m
The forces can be shown in the figure as follows:

At equilibrium,
Surface normal reaction force, N = mgcos37°
Frictional force, f = μN where, μ is the coefficient of friction
⇒ f = mgsin37°
Net force acting on the block, F = mgsin37° - f
⇒ F = mgsin37° - μmgcos37°
⇒ F = mg(sin37° - μcos37°)
At equilibrium, work done by the block is equal to the potential energy of the spring according to law of conservation of energy.
So, mg(sin37° - μcos37°)s = (1/2)ks2
⇒ 1 kg×9.8 m s-2×(0.602 – μ0.799)×0.1 = (1/2)×100Nm-1×(0.1)2
⇒ 0.602 - μ × 0.799 = 0.51
⇒ μ × 0.799 = 0.092
⇒ μ = 0.115