In Millikan’s oil drop experiment, what is the terminal speed of an uncharged drop of radius 2.0 × 10–5 m and density 1.2 × 103 kg m–3. Take the viscosity of air at the temperature of the experiment to be 1.8 × 10–5 Pa s. How much is the viscous force on the drop at that speed? Neglect buoyancy of the drop due to air.
Radius of the drop = 2 × 10-5 m
Density of the drop = 1.2 × 103 kg/m3
Viscosity of the air, μ = 1.8 × 10-5 Pa.s
*Consider density of air to be zero in order to neglect buoyancy force create by air on drop.
Terminal velocity is given by the relation,

Where,
r = radius
ρ = highest density = density of drop
ρ0 = Lowest density = density of air
g = acceleration due to gravity = 9.81 m/s
μ = viscosity
∴ 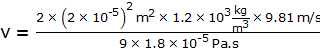
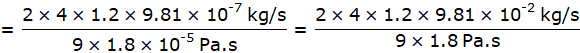
= 5.8 × 10-2 m/s
= 5.8 cm/s
Hence, the terminal speed of the drop = 5.8 cm/s
The viscous force on the drop is given by,
![]()
Where,
μ = viscosity
r = radius
v = velocity
∴ ![]()
![]()
= 3.9 × 10-10 N
Hence, the viscous force on drop = 3.9 × 10-10 N