What is the energy in joules, required to shift the electron of the hydrogen atom from the first Bohr orbit to the fifth Bohr orbit and what is the wavelength of the light emitted when the electron returns to the ground state? The ground state electron energy is –2.18 × 10–11 ergs.
Given:
Ground State Electron energy = –2.18 × 10–11 ergs
Finding Energy:
To convert energy from ergs to joules
1 ergs is equal to 10-7 Joules
So –2.18 × 10–11 ergs = –2.18 × 10–11 × 10-7
So, Ground State Electron energy = = –2.18 × 10–18 J
Energy to shift the electron from n = 1 to n= 5 state is given by following relation:
Eh = E5 – E1
The energy of hydrogen atom is given by the following equation:
En = -2n2me4Z2/n2h2
Where
m = mass of electrons
Z = atomic mass of atom
E = charge of electron
h = Planck’s constant
So, the energy required is = 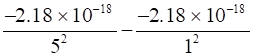
= 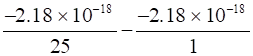
= 2.0928 × 10–18 J
Therefore, the energy to shift the electron from n=1 to n=5 state is 2.093 × 10–18 J.
Finding Wavelength:
By Planck’s relation we have,
Energy, E = h×v
But we know v = [c] / [λ]
Where
c = Speed of Light
v= Frequency
λ = Wavelength
So E = hc /λ
λ = hc / E
= [[6.626×10-34] × [3×108]] / [2.0928 × 10–18]
= [1.9878×10-25] / [2.0928 × 10–18]
= 9.498×10-8 m
Therefore, the wavelength is 9.5×10-8 m