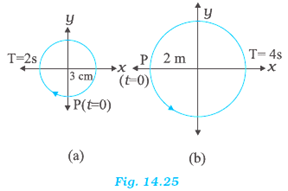
Figures 14.25 correspond to two circular motions. The radius of the circle, the period of revolution, the initial position, and the sense of revolution (i.e. clockwise or anti-clockwise) are indicated on each figure. Obtain the corresponding simple harmonic motions of the x-projection of the radius vector of the revolving particle P, in each case.

Question

Philoid · Accepted Answer

We know as a particle move in circular path the projection on x and y axis of displacement covered by particle represent a simple harmonic motion as the particle moves the angle subtended by it changes and if velocity is uniform its angular velocity or rate of change of angle subtended at centre with respect to original position become constant (a fixed value), projections can be represented as a function of sine and cosine in terms of angular velocity or time period and time

(a) Here particle started from a point on negative Y axis and started moving in a circular path in clockwise direction, now the time period of one complete rotation is given as T = 2s, so the particle will return to its original position in 2s, so after 1 second it will be on diametrically opposite point

Now let angle made by line joining particle to centre make an angle