Using section formula, show that the points A (2, –3, 4), B (–1, 2, 1) and 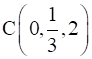 are collinear.
are collinear.
Let the point P divides AB in the ratio k : 1.
Then,
Comparing this information with the details given in the question, we have
x1 = 2, y1 = -3, z1 = 4; x2 = -1, y2 = 2, z2 = 1 and m = k, n = 1
By section formula,
We know that the coordinates of the point R which divides the line segment joining two points P (x1, y1, z1) and Q (x2, y2, z2) internally in the ratio m : n is given by:
![]()
So, we have,
The coordinates of P = ![]()
Now, we check if for some value of k, the point coincides with the point C.
Put ![]()
⇒ -k + 2 = 0 ⇒ k = 2
When k =2, then ![]()
And ![]()
Therefore, C ![]() is a point which divides AB in the ratio 2 : 1 and is same as P.
is a point which divides AB in the ratio 2 : 1 and is same as P.
Hence, A, B, C are collinear.
4