Which of the following statements are true and which are false? In each case give a valid reason for saying so. (i) p: Each radius of a circle is a chord of the circle. (ii) q: The centre of a circle bisects each chord of the circle. (iii) r: Circle is a particular case of an ellipse. (iv) s: If x and y are integers such that x y, then –x

Question

Which of the following statements are true and which are false? In each case give a valid reason for saying so. (i) p: Each radius of a circle is a chord of the circle. (ii) q: The centre of a circle bisects each chord of the circle. (iii) r: Circle is a particular case of an ellipse. (iv) s: If x and y are integers such that x > y, then –x < – y. (v) t : √11 is a rational number.

Philoid · Accepted Answer

(i)

This statement is false

A chord intersect the circle in two points.

OB & OC are radius of circle

But they don't intersect the circle at two points

Hence OB & OC are not the Chord of a circle.

(ii)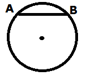

The given statement is false

Here AB is a chord as it intersect the circle at two points,

But it does not pass through center O.

So, center of circle does not bisect each chord of the circle.

(iii) This statement is true

Equation of ellipse is

putting a = b

⇒ x² + y² = b²

which is the equation of circle.

So, circle is the particular case of ellipse.

(iv) x>y

Multiplying – 1 both sides

(– 1)x<(– 1)y

⇒ – x < – y

If – 1 is multiplied to both L.H.S & R.H.S, sign of inequality changes.

This is the rule of inequality

Hence the given statement is true.

(v) cannot be written in the form of p/q

Hence is irrational.

Hence the given statement is false.