As we know that the polar representation of a complex number z = x + iy is

z = r (cos θ + i sin θ) where is the modulus of the complex number and θ is the argument of the complex number, denoted by arg z.

So, now, let –1 = r cos θ and –√3 = r sin θ ……….(i)

Squaring both sides, we get

1 = r² cos² θ and 3 = r² sin² θ

Adding both the equations, we get

1 + 3 = r² cos² θ + r² sin² θ

⇒ 4 = r² (cos² θ + sin² θ)

⇒ 4 = r² or r² = 4 [∵ sin² θ + cos² θ = 1]

⇒ r = √4

⇒ r = 2 (conventionally, r>0) ……….(ii)

Substituting r = 2 in (i), we get

–1 = 2 cos θ and –√3 = 2 sin θ

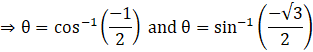
∵ We know that the complex number –1 – i √3 lies in the third quadrant and the value of the argument lies between - π and π, i.e. - π < θ ≤ π.

……….(iii)

From (ii) and (iii), we have

r = 2 and