Which of the following cannot be valid assignment of probabilities for outcomes of sample Space ![]()
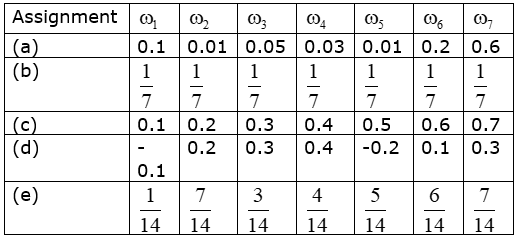
a) Both the conditions of axiomatic approach hold true in the given assignment, that is
1) Each of the number p(wi) is less than zero and is positive
2) Sum of probabilities is
0.01 + 0.05 + 0.03 + 0.01 + 0.2 + 0.6 = 1
The given assignment is valid.
b) Both the conditions of axiomatic approach hold true in the given assignment, that is
1) Each of the number p (wi) is less than zero and is positive
2) Sum of probabilities is
![]()
The given assignment is valid.
c) Both the conditions of axiomatic approach in the given assignment are
1) Each of the number p(wi) is less than zero and is positive
2) Sum of probabilities is
0.1 + 0.2 + 0.3 + 0.4 + 0.5 + 0.6 + 0.7 = 2.8 > 1
So, the 2nd condition is not satisfied
Which states that p(wi) ≤ 1
The given assignment is not valid.
d) The conditions of axiomatic approach don’t hold true in the given assignment, that is
1) Each of the number p(wi) is less than zero but also negative
To be true each of the number p(wi) should be less than zero and positive
So, the assignment is not valid
e) Both the conditions of axiomatic approach in the given assignment are
1) Each of the number p(wi) is less than zero and is positive
2) Sum of probabilities is
![]()
The second condition doesn’t hold true so the assignment is not valid.