A farmer moves along the boundary of a square field of side 10 m in 40 s. What will be the magnitude of displacement of the farmer at the end of 2 minutes 20 seconds from his initial position?
First we will convert the time into seconds
Total time = 2 minutes 20 seconds
= 2× 60 seconds + 20 seconds
= 120 seconds + 20 seconds
= 140 seconds
Now, If in 40 s,
Number of rounds made = 1
So, In 140 sec the number of rounds made
= 1/40×140
= 3.5 rounds
Thus, the farmer will make 3.5 rounds of the square field.
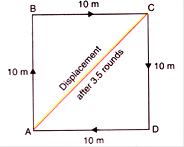
If the farmer starts from position A (see figure), then after three complete rounds, he will reach at starting position A.
But in the next half round, the farmer will move from A to B, and B to C, so that his final position will be at C.
Hence, the total displacement of the farmer will be AC.
Now, ABC is a right angled triangle with AC as hypotenuse.
So,
(AC)2 = (AB)2 + (BC)2
(AC)2 = (10)2 + (10)2
(AC)2 = 100 + 100
(AC)2 = 200
AC = 14.143 m
Hence, the magnitude of displacement of the farmer at the end of 2 minutes and 20 seconds will be 14.143 metres.