Mathematics Part-II

Book: Mathematics Part-II

Chapter: 11. Three Dimensional Geometry

Subject: Maths - Class 12th

Q. No. 5 of Miscellaneous Exercise

Listen NCERT Audio Books - Kitabein Ab Bolengi

5

If the coordinates of the points A, B, C, D be (1, 2, 3), (4, 5, 7), (–4, 3, –6) and (2, 9, 2) respectively, then find the angle between the lines AB and CD.

Angle between the lines with direction ratios a1, b1, c1 and a2, b2, c2 is given by


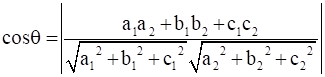
A line passing through A(x1, y1, z1) and B(x2, y2, z2) has direction ratios (x1 - x2), (y1 - y2), (z1 - z2)


Direction ratios of line joining the points A(1,2,3) and B(4,5,7)


= (4 - 1), (5 - 2), (7 - 3)


= (3,3,4)


∴ a1 = 3, b1 = 3, c1 = 4


Direction ratios of line joining the points C(-4, 3, -6) and B(2,9,2)


= (2 - (-4)), (9 - 3), (2-(-6))


= (6,6,8)


∴ a2 = 6, b2 = 6, c2 = 8


Now,







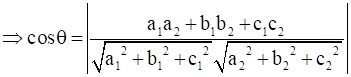
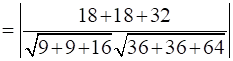
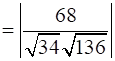
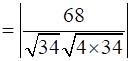
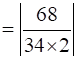∴ cosθ = 1


So, θ = 0°


Hence, Angle between the lines AB and CD is 0°.


5

Chapter Exercises

Exercise 11.1
Exercise 11.2
Exercise 11.3
Miscellaneous Exercise

More Exercise Questions

1

Show that the line joining the origin to the point (2, 1, 1) is perpendicular to the line determined by the points (3, 5, –1), (4, 3, –1).
2

If l1, m1, n1 and l2, m2, n2 are the direction cosines of two mutually perpendicular lines, show that the direction cosines of the line perpendicular to both of these are (m1n2 - m2n1), (n1l2 - n2l1), (l1m2 - l2m1)
3

Find the angle between the lines whose direction ratios are a, b, c and b – c, c – a, a – b.
4

Find the equation of a line parallel to x - axis and passing through the origin.
5

If the coordinates of the points A, B, C, D be (1, 2, 3), (4, 5, 7), (–4, 3, –6) and (2, 9, 2) respectively, then find the angle between the lines AB and CD.
6

If the lines and are perpendicular, find the value of k.
7

Find the vector equation of the line passing through (1, 2, 3) and perpendicular to the plane .
8

Find the equation of the plane passing through (a, b, c) and parallel to the plane .
9

Find the shortest distance between lines

and .
10

Find the coordinates of the point where the line through (5, 1, 6) and (3, 4,1) crosses the YZ - plane.
11

Find the coordinates of the point where the line through (5, 1, 6) and (3, 4, 1) crosses the ZX - plane.
12

Find the coordinates of the point where the line through (3, –4, –5) and (2, –3, 1) crosses the plane 2x + y + z = 7.
13

Find the equation of the plane passing through the point (–1, 3, 2) and perpendicular to each of the planes x + 2y + 3z = 5 and 3x + 3y + z = 0.
14

If the points (1, 1, p) and (–3, 0, 1) be equidistant from the plane , then find the value of p.
15

Find the equation of the plane passing through the line of intersection of the planes and and parallel to x-axis.
16

If O be the origin and the coordinates of P be (1, 2, –3), then find the equation of the plane passing through P and perpendicular to OP.
17

Find the equation of the plane which contains the line of intersection of the planes and . And which is perpendicular to the plane .
18

Find the distance of the point (–1, –5, –10) from the point of intersection of the line and the plane .
19

Find the vector equation of the line passing through (1, 2, 3) and parallel to the planes s and .
20

Find the vector equation of the line passing through the point (1, 2, – 4) and perpendicular to the two lines:

and .
21

Prove that if a plane has the intercepts a, b, c and is at a distance of p units from the origin, then

22

Distance between the two planes: 2x + 3y + 4z = 4 and 4x + 6y + 8z = 12 is
23

The planes: 2x – y + 4z = 5 and 5x – 2.5y + 10z = 6 are