A driver of a car travelling at 52 km h-1 applies the brakes and accelerates uniformly in the opposite direction. The car stops in 5 s. Another driver going at 34 km h-1 in another car applies his breakes slowly and stops in 10 s. On the same graph paper, plot the speed versus time graphs for the two cars. Which of the two cars travelled farther after the brakes were applied?
Firstly, for the first car:
Initial speed, u = 52 km/h2
= 
= 14.4 m s-1 (i)
Final speed, v = 0 (As the car stops) (ii)
Time taken, t = 5 s (iii)
Now, for the second car:
Initial speed, u = 34 km/h
= 
= 9.4 m/s (iv)
Final speed, v = 0 (As the car stops) (v)
Time taken, t = 10 s (vi)
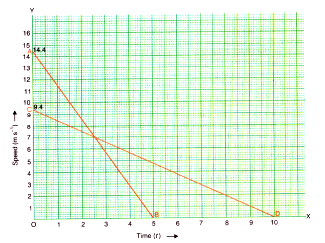
In the graph, we will plot time taken in X-axis and speed in the Y-axis. In the graph the sloping point AB is the speed-time graph for the first car and the sloping point CD is the speed-time graph for the second car.
Now we have to find the distance travelled under the graph line AB = Area under triangle OAB
= 
× base × height
= 
× 5 × 14.4
= 36 m (vii)
Now we will find the distance travelled under the line CD = Area under triangle OCD
= 
× base × height
= 
× 10 × 9.4
= 47 m (viii)
Therefore, from (vii) and (viii) it is clear that the second car travels farther as compared to the first after the application of brakes.