Figure shows the distance-time graphs of three objects A, B and C. Study the graphs and answer the following questions:
(a) Which of the three is travelling the fastest?
(b) Are all three ever at the same point on the road?
(c) How far has C travelled when B passes A?
(d) How far has B travelled by the time it passes C?
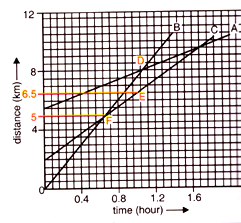
(a) The slope of distance time graph of the moving objects represents the speed of those objects.
Greater the slope of an object, higher is its speed.
Now, in the given figure we can see that the slope of distance time graph of object B is the maximum, hence object B has the maximum speed.
In other words the object B is travelling with the fastest speed.
(b) In order to be at the same point on the road, the respective distance and time values for all the three moving objects need to be the same. Since the distance time graph lines of the three objects A, B, and C do not cross at any point, therefore, the three objects are never at the same point on the road.
(c) We can see from the given figure that when B passes A at point D, then the C is at point E.
So, if we locate the distance corresponding to point E on the Y-axis, we would find that it is 6.5 km.
Thus, C has travelled 6.5 km when B passes A.
(d) The distance time graphs of B and C meet at point F.
If we locate the distance corresponding to point F on the Y-axis, we will find that it is 5 km.
Thus, B has travelled 5 km by the time it passes C.