The following is the distance time table of an object in motion:
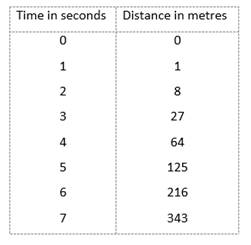
(a) What conclusion can you draw about the acceleration? Is it constant, increasing, decreasing or zero?
(b) What do you infer about the forces acting on the object?
(a) Given,
Time (t) = 0 s
Distance (s) = 0 m
And at time, (t) = 1 s
Distance (s) = 1 m
When time (t) = 2 s
Distance (s) = 8 m
We know that,
(2)3 = 2×2×2 = 8
When time (t) = 3 s,
Distance (s) = 27 m
(3)3 = 3×3×3 = 27
From above, we can conclude that:
Distance ∝ (Time)3
s ∝ t3
(i) When the distance travelled is proportional to time (s ∝ t), then the object has constant velocity.
Hence, acceleration in that case would be zero.
Since, s ∝ t3 (So the acceleration in this case cannot be zero)
(ii) When the distance travelled is proportional to the square of time (s∝t2), then the object has constant acceleration.
Since, s∝t3 (So the acceleration in this case cannot be constant)
(iii) The data given in this question shows that the distance travelled is proportional to the cube of time (s∝t3), therefore, the conclusion drawn is that the acceleration is increasing uniformly with time.
(b) We know that,
Force = Mass × Acceleration
F= m × a
In other words we can also say that the acceleration of a body is proportional to the force applied to it. Now, since the acceleration of the object in this case is increasing uniformly with respect to time, therefore, the forces acting on the object must also be increasing uniformly with time.