The weight of a body is the force acting one it due to earth’s gravity and is the product of mass and acceleration due to gravity is given as

W = mg

Where W is the weight of a body of mass m on the surface of the earth, g is acceleration due to gravity on the surface of the earth

Now as the height from the surface of the earth increases acceleration due to gravity decreases, so the weight of the body also decreases i.e.

W_h = mg_h

Where W_h is the weight of a body of mass m kept on h height above the surface of the earth, Let g_h be the acceleration due to gravity at a height h above the surface of the earth

Now acceleration due to gravity above surface h varies according to the relation

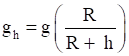
Here,

g_h is the acceleration due to gravity at a height h above the surface of the earth, g is acceleration due to gravity on earth’s surface and R is Radius of the Earth

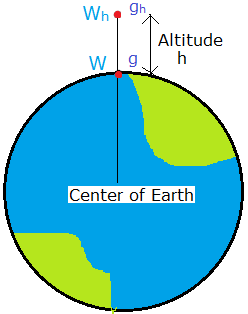
The situation has been shown in figure

In this case, height is equal to half of the radius of earth i.e.

H = R/2

Therefore,

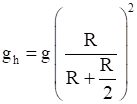
On solving further we get

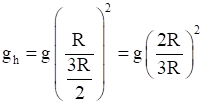
i.e. we get

g_h = 4/9 g

So acceleration due to gravity at a height R/2 above the surface of the earth is 4/9 times to that on the surface of the earth

So weight at that height will be

W_h = m(4/9g) = 4/9 mg

i.e.

W_h = 4/9 W

So weight will be 4/9 times weight at the surface of the earth

We are given weight on the surface of the earth as

W = 63 N, so we have

W_h = 4/9 × 63N = 28 N

So the weight of the body or gravitational force on it due to the earth at a height equal to half the radius of the earth will be 28 N