Rocket fired from surface of Earth and will have kinetic energy(Positive) by virtue of speed which is taking it away from surface of Earth, and gravitational potential energy(Negative) is trying to pull it towards the centre of Earth, total energy of the rocket is the sum of kinetic energy and gravitational potential energy , and total energy is conserved , i.e. will remain same

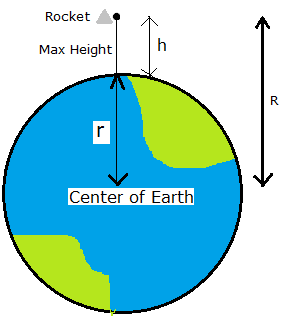
Now as rocket is fired upward Earth’s gravity will apply force on it pull it back, and speed of rocket will decrease and kinetic energy also, potential energy on another hand will increase and become less negative and at a maximum height h above the surface of Mars at a distance R from centre of mars of radius r, all kinetic energy will be converted to potential energy, and rocket will come to rest and then will revert back towards surface of Mars

The situation has been shown in figure

We know Kinetic Energy is given as

Where K is the kinetic energy of a body of mass moving with speed v, here we are given

The initial speed of Rocket as

v = 5 km s^–1 = 5000 ms^-1

let Mass of the rocket be m

so kinetic energy is

Now Gravitational potential energy is negative and decreases as we move away from System and at an infinite distance from the planet

Now Gravitational potential at a point is given by the relation

U = -GMm/R

Where U is the potential Energy of a body of mass m at a point at a distance R from a center of mass of Body of mass M, G is universal Gravitational constant

Initially, Rocket is at the surface of Earth so its distance from the centre of mars is equal to the radius of Earth i.e.

R = r = 6400 km = 6.4 × 10⁶ m

let Mass of the rocket be m

mass of planet Earth is

M = 6×10²⁴ kg

Value of universal gravitational constant

G = 6.67 × 10^-11 Nm²Kg^-2

So putting these value we get initial potential energy U_i as

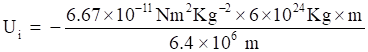
= -6.25m × 107 J

Now finally after reaching a height h, the rocket will come to rest and its velocity will be 0, i.e.

v = 0 m/s

so final kinetic energy will also be zero i.e.

K_f = 0 J

Now after reaching a height h, the distance of rocket from the centre of mars will be

R = r + h

So final potential energy U_f will be

i.e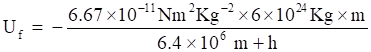

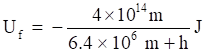
The total energy of a body is the sum of kinetic energy and potential energy

T = K + U

Where T is the total energy, U is potential energy and K is kinetic energy

So total initial energy will be

T_i = U_i + K_i

i.e. T_i = -6.25m × 10⁷ J + 25m/2 × 10⁶ J

= - 50.0m × 10⁶ J

So Total Final Energy will be

T_f = U_f + K_f

i.e.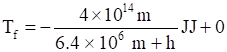

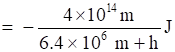
Now by the law of conservation of energy, we know the total initial energy of rocket must be equal to the total final energy of the rocket i.e.

T_i = T_f

i.e.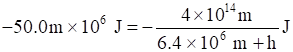

now cancelling out a negative sign and term m from both sides and solving we get

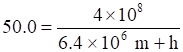After cross multiplication we get

320 × 10⁶ + 50h = 4 × 10⁸

i.e. 50h = 400 × 10⁶ – 320 × 10⁶ = 80 × 10⁶

so we get h as

h = 80 × 10⁶/50 = 1.6 × 10⁶ m

So the rocket will reach a height of 1.6 × 10⁶ m above the surface of Earth

Its Distance from centre of earth will be

R = r + h

Where r is the radius of earth which is

r = 6.4 × 10⁶ m

so we get

R = 6.4 × 10⁶ m + 1.6 × 10⁶ m

= 8 × 10⁶ m

So satellite is at a distance of 8 × 10⁶ m from surface of earth