Now let us assume both stars to be initially at rest and come towards each other due to the gravitational force of attraction and as they come closer their speed keeps on increasing, suppose they are initially at a distance of d

d = 10⁹ km = 10¹² m

For head-on collision, the least distance between the stars will be equal to twice of their radius

The Initial situation and when they are just going to collide has been displayed in the figure below

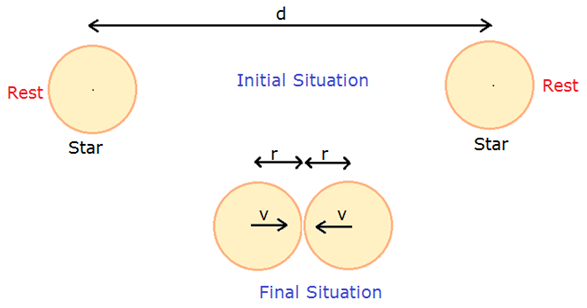
For head-on collision, the least distance between the stars will be equal to twice of their radius, we are given the radius of each star as

r = 10⁴ km = 10⁷ m

so least distance or final between the stars will be

d’ = 2r = 2 × 10⁷ m

we will use the law of conservation of energy

The total energy of a body is the sum of kinetic energy and potential energy

T = K + U

Where T is the total energy, U is potential energy and K is kinetic energy

We know Kinetic Energy is given as

Where K is the kinetic energy of a body of mass moving with speed v and potential energy is given as

U = -Gm₁m₂/R

Where U is the potential Energy of a body of mass m₁ at a point at a distance R from a center of mass of Body of mass m₂, G is universal Gravitational constant

Initially, Both the Stars are assumed to be at rest i.e their speed is

v = 0

so initial Kinetic energy of both the stars will be zero so the total kinetic energy of the system consisting two stars will also be zero

i.e. K_i = 0

Initially, potential energy of the system will have a maximum value when the separation between stars will be maximum

now masses of both the stars are equal i.e.

m¹ = m² = m = 2 × 10³⁰ kg

value of the universal gravitational constant is

G = 6.67 × 10^-11 Nm²Kg^-2

And the initial separation between the stars is

R = d = 10¹² m

so the initial gravitational potential energy of the system U_i will be

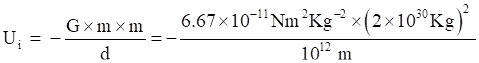
= -26.68 × 10³⁷ J

So total initial energy of the system will be

T_i = U_i + K_i

i.e. T_i = -26.68 × 10³⁷ J + 0 J

= -26.68 × 10³⁷ J

Now finally let us assume both stars gained speed v moving towards each other and are about to collide, so the final kinetic energy of the system will be equal to the sum of kinetic energy of both the stars i.e.

K_f = 1/2 mv² + 1/2 mv² = mv²

Where m is the mass of each star, we know

m = 2 × 10³⁰ kg

so the final kinetic energy of the system will be

K_f = 2 × 10³⁰ × v²

Finally when stars will come close and separation between them will be minimum potential energy will also be at its minimum value

We know final separation between the stars is

R = d’ = 2 × 10⁷ m

now masses of both the stars are equal i.e.

m₁ = m₂ = m = 2 × 10³⁰ kg

value of the universal gravitational constant is

G = 6.67 × 10^-11 Nm²Kg^-2

so Final gravitational potential energy of the system U_f will be

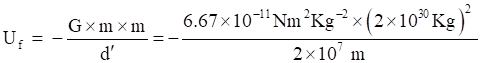
= -13.34 × 10⁴² J

So total final energy of the system will be

T_f = U_f + K_f

i.e. T_f = -13.34 × 10⁴² J + 2 × 10³⁰ × v²

NOTE: while calculating total kinetic energy of the system we added individual kinetic energies of both the stars but we did not do this for potential energy as potential energy of a body due to another body is the potential energy of the system and taking any body as reference (P.E. of star 1 w.r.t star 2 or P.E. of star 2 w.r.t. star 1) when we calculate potential energy value is same

now as we can observe potential energy of the system has been lost and is converted to kinetic energy but according to the law of conservation of energy total energy will be same i.e.

T_i = T_f

So equating values

-26.68 × 10³⁷ J = -13.34 × 10⁴² J + 2 × 10³⁰ Kg × v²

Solving further

2 × 10³⁰ Kg × v² = 13.34 × 10⁴² J – 0.00026 × 10⁴² J

v² = 6.66 × 10¹² m²s^-2

i.e

So, we get final speed of both the stars as 2.58 × 10⁶ ms^-1