Let us consider two spheres each of mass M and radius r are placed at a distance d apart from each other on a horizontal table,

Here a mass of both the spheres is

M = 100 Kg

Here Radius of both the spheres is

r = 0.10 m

The separation between both the spheres is

d = 1.0 m

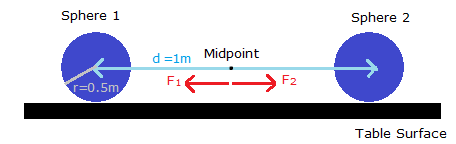
suppose sphere 1 is on left side of table and Sphere 2 is on right side of table, suppose a body is placed at the midpoint of line joining two spheres, Since gravitational force is always attractive in nature so let Gravitational Force due to sphere 1 be F₁ and it will be in left direction, and force due to sphere 2 is F₂ and will act on right side

The situation has been shown in the figure

We know gravitational force on a body is given as

Where F is the gravitational force

G is universal gravitational Constant

G = 6.67 × 10^-11 Nm²Kg^-2

M is mass of the first body

m is the mass of the second body

and R is the distance between the two bodies

now here if Body is kept at the midpoint of the line joining two spheres then the separation between a body at the midpoint and the two spheres will be same and equal to half the distance between two spheres suppose R₁ is a distance of the first sphere from midpoint and R₂ is a distance of the second sphere from midpoint then we have

R₁ = R₂ = R = d/2 = 1/2 = 0.5m

now we get that magnitude of force on a body kept at midpoint by sphere 1 and sphere 2 is same as for both the forces F₁ and F₂, mass of first body(Sphere) M, mass of the second body(body at midpoint) m, separation between both the bodies R is same and Universal gravitational constant is same , i.e. magnitude of forces

F₁ = F₂ = GMm/R²

Now magnitude of both the forces will be exactly same but directions will be opposite, and force is a vector quantity, so forces will be added vectorially and we know Force of equal magnitude and opposite direction result in Zero as both cancel out each other, Magnitude of the net force is

F = F₁ – F₂ = 0 N

So net force at the centre is 0 N

Now Gravitational potential at a point is given by the relation

V = -GM/R

Where V is the potential of a point at a distance R from a Body of mass M, G is universal Gravitational constant

Potential is a scalar quantity, so it does not have a direction and is added directly not net potential at the centre will be

V = V₁ + V₂

Where V₁ is the potential due to the first body and V₂ is the potential due to the second body

We know potential due to the first sphere will be given as

V₁ = -GM₁/R₁

Where M1 is the Mass of the first sphere

M₁ = M = 100 Kg

R₁ is a distance of the first sphere from Midpoint

R₁ = R = 0.5 m

G is universal gravitational Constant

G = 6.67 × 10^-11 Nm²Kg^-2

Now putting the values in above equation, we get

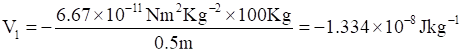
similarly, potential due to the second sphere will be given as

V₂ = -GM₂/R₂

Where M₂ is the Mass of the Second sphere

M₂ = M = 100 Kg

R₂ is a distance of the Second sphere from Midpoint

R₂ = R = 0.5 m

G is universal gravitational Constant

G = 6.67 × 10^-11 Nm²Kg^-2

Now putting value we get

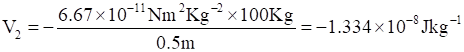
So net potential at mid-point is

V = V₁ + V₂

= -1.334 × 10^-8 Jkg^-1 + (-1.334 × 10^-8 Jkg^-1)

= -2.668 × 10^-8 Jkg^-1

If a body is placed at Midpoint it will be in equilibrium as net force acting on it will be Zero , but it be in unstable equilibrium as if it is displaced slightly to left or right it will be pulled towards the sphere which is at less distance from it and not come back to its mean position this will happen because gravitational force of attraction is inversely proportional to distance so as distance between the two bodies decrease force of attraction increase and vice versa, so on even slight displacement force on body due to one sphere will increase and decrease due the other sphere which is at a greater distance and it will be pulled towards body at lesser distance and never come back to original position such an equilibrium condition is called unstable equilibrium.