Gravitational potential energy is the energy possessed by a body at some point due to its mass and its power to attract object due to its Gravity

We know Gravitational potential at a point is given by the relation

V = -GM/R

Where V is the potential of a point at a distance R from a Body of mass M, G is universal Gravitational constant

Now R is the Distance from the center of the body, so in case of a satellite at some height h from earth surface, the distance of earth’s satellite from the centre of earth R will be equal to sum of earth’s radius r and height from the surface of earth h

R = r + h

The situation has been shown in figure

We are given Radius of earth

r = 6400 Km

the height of satellite from Earth’s Surface

h = 36,000 km

so the net distance of point where the satellite is located to the center of the earth is

R = r + h = 6400 Km + 36,000 km

= 42400 Km = 4.24 × 10⁷ m

Mass of earth

M = 6.0 × 10²⁴ kg

Value of universal gravitational Constant

G = 6.67 × 10^-11 Nm²Kg^-2

Now putting the values in above equation we get potential at the point as

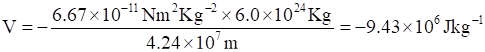
So the potential due to earth’s gravity at the site of this satellite is

-9.43 × 10⁶ Jkg^-1