Every Object possesses two kinds of energy potential energy due to its position and kinetic energy due to its speed, Whenever a body is bound to a system or another body like in this case Spaceship is bound to the solar system due to gravitational force applied by it in order to keep Spaceship inside the solar system , the total energy of such a object will be negative, whenever total energy of object will become positive or even zero it will escape from that system irrespective of all the forces being applied to it by the system.

The total energy of a body is the sum of kinetic energy and potential energy

T = K + V

Where T is the total energy, V is potential energy and K is kinetic energy kinetic energy of a body is always positive and depends upon the speed of the body, Gravitational potential energy is negative and decreases as we move away from System and at an infinite distance from the planet

Kinetic Energy is given as

Where K is the kinetic energy of a body of mass m, moving with velocity v

The potential energy of a body above the surface of the earth is given as

V = -GMm/r

Where V is the gravitational Potential Energy of body of mass m at a distance r from the centre of a body of mass M, G is universal gravitational Constant

Now here we are considering system to consist only of sun and Mars as all other bodies in solar system have very less mass compared to these, so total gravitation potential energy of body will sum of gravitational potential energy due to sun and due to Mars, now spaceship is on surface of Mars so its distance from surface of Mars will equal to radius of mars r, and its distance from sun will nearly be equal to distance of mars from sun i.e. radius of Mars orbit

As can be seen in the figure

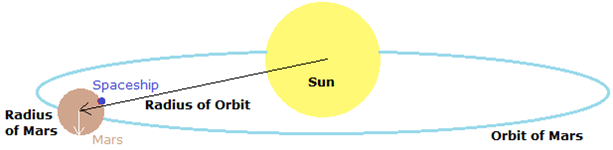
So if we let the mass of spaceship be m and mass of mars be Mm then and radius of mars be r, then gravitational potential energy of object due to mars will be

V_m = -GMm_m/r

Similarly, mass of Sun be M_s then and radius of orbit of mars be R, then gravitational potential energy of object due to Sun will be

V_s = -GMs_m/R

So adding both potential energies we get total potential energy of the spaceship as

V = V_s + V_m

i.e. V = -GM_sm/R + (-GM_mm/r)

= -GM_sm/R -GM_mm/r = -Gm(M_s/R + M_m/r)

Spaceship is assumed to be at rest initially so its speed will be 0, i.e. v = 0 m/s

So its kinetic energy will be zero, so its total energy will be its total potential energy

so we need to give it energy which will increase its speed and increase its kinetic energy and total energy will be positive, if we supply E amount of energy so total energy of spaceship will be

T = E + V

Or we get

T = E + [-Gm(M_s/R + M_m/r)]

If Spaceship has to move out of System’s influence i.e. move out of the solar system its total energy should be positive i.e.

T ≥ 0

So taking the condition for spaceship to just escape the solar system

T = 0

i.e. E + [-Gm(M_s/R + M_m/r)] = 0

i.e. we get total energy that need to be supplied to the space ship is

E = Gm(M_s/R + M_m/r)

Now we know value of universal gravitational constant as

G = 6.67 × 10^-11 Nm²Kg^-2

Mass of the space ship is

m = 1000 kg

Mass of the sun is

M_s = 2 × 10³⁰ kg

mass of mars is

M_m = 6.4 × 10²³ kg

Radius of mars is

r = 3395 km = 3.395 × 10⁶ m

Radius of the orbit of mars is

R = 2.28 × 10⁸ km = 2.28 × 10¹¹ m

so putting the values of G, r, R, m, M_s, M_m in above equation we get

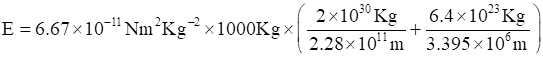
= 6.67 × 10^-11Nm²Kg^-2 × 1000Kg × (8.77 × 10¹⁸kgm^-1 + 1.88 × 10¹⁷ kgm^-1)

= 6.67 × 10^-8 Nm²Kg^-1 × 8.95 × 10¹⁸ kgm^-1

= 5.98 × 10¹¹ J

So, to launch the spaceship out of the solar system we need to supply 5.98 × 10¹¹ J of energy to Spaceship