Let us assume the remaining two observations be x and y

The given observations in the question are 2, 4, 10, 12, 14, x, y

x + y = 14 (i)

It is also given in the question that,

Variance = 16

We know that,

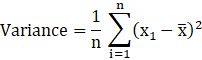
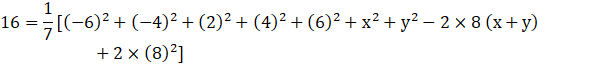
x² + y² = 112 - 12

x² + y² = 100 (ii)

Thus, by using (i) we have:

x² + y² + 2xy = 196 (iii)

Now, from equation (ii) and (iii) we have:

2xy = 196 – 100

2xy = 96 (iv)

Now subtracting equation (iv) from (ii), we get:

x² + y² – 2xy = 100 – 96

(x – y)² = 4

x – y = 2 (v)

Hence, from equation (i) and (v) we have:

When x – y = 2 then x = 8 and y = 6

And, when x – y = - 2 then x = 6 and y = 8

∴ The remaining observations are 6 and 8