An aeroplane can carry a maximum of 200 passengers. A profit of Rs 1000 is made on each executive class ticket and a profit of Rs 600 is made on each economy class ticket. The airline reserves at least 20 seats for executive class. However, at least 4 times as many passengers prefer to travel by economy class than by the executive class. Determine how many tickets of each type must be sold in order to maximise the profit for the airline. What is the maximum profit?
let x and y be the number of tickets of executive class and economy class respectively. Obviously x ≥ 0, y ≥ 0. Mathematical formulation of given problem is as follows:
Maximize Z = 1000x + 600y ….(1)
Subject to the constraints,
x + y ≤ 200 …..(2)
x ≥ 20 …..(3)
y - 4x ≥ 0 ….(4)
x ≥ 0, y ≥ 0 ….(5)
Now let us graph the feasible region of the system of inequalities (2) to (5). The feasible region (shaded) is shown in the fig. Here, we can observe that the feasible region is bounded.
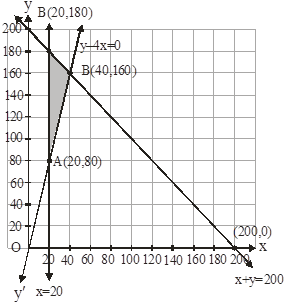
The coordinates of the corner points A(20,80),B(40,160) and C(20,180).
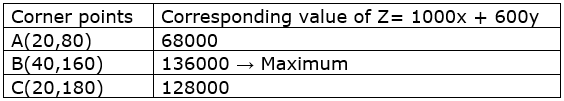
Now, we find the maximum value of Z. According to table the maximum value of Z = 136000 at point B (40,160).
Hence, 40 and 160 be the number of tickets of executive class and economy class respectively to get the maximum profit.