Two godowns A and B have grain capacity of 100 quintals and 50 quintals respectively. They supply to 3 ration shops, D, E and F whose requirements are 60, 50 and 40 quintals respectively. The cost of transportation per quintal from the godowns to the shops are given in the following table:
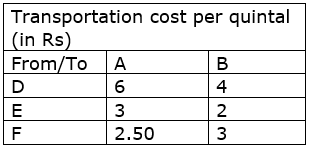
How should the supplies be transported in order that the transportation cost is minimum? What is the minimum cost?
let x and y quintal of grain supplied by A to shop d and E respectively. Then 100 - x - y will be supplied to shop F. Obviously x ≥ 0, y ≥ 0.
Since requirement at shop D is 60 quintal but supplied x quintal hence, 60 - x is sent form B to D.
Similarly requirement at shop E is 50 quintal but supplied y quintal hence, 50 - y is sent form B to E.
Similarly requirement at shop E is 40 quintal but supplied 100 - x - y quintal hence, 40 - (100 - x - y) i.e. x + y - 60 is sent form B to E.
Diagrammatically it can be explained as:

As x ≥ 0, y ≥ 0 and 100 - x - y ≥ 0 ⇒ x + y ≤ 100
60 - x ≥ 0, 50 - y ≥ 0 and x + y - 60 ≥ 0
⇒ x ≤ 60, y ≤ 50 and x + y ≥ 60
Total transportation cost Z can be calculated as:
Z = 6x + 3y + 2.5(100 - x - y) + 4(60 - x) + 2(50 - y) + 3(x + y - 60)
= 6x + 3y + 250 - 2.5x - 2.5y + 240 - 4x + 100 - 2y + 3x + 3y - 180
Z = 2.5x + 1.5y + 410
Mathematical formulation of given problem is as follows:
Minimize Z = 2.5x + 1.5y + 410 ….(1)
Subject to the constraints,
x + y ≤ 100 …..(2)
x ≤ 60 …..(3)
y ≤ 50 ….(4)
x + y ≥ 60 …(5)
x ≥ 0, y ≥ 0 ….(6)
Now let us graph the feasible region of the system of inequalities (2) to (6). The feasible region (shaded) is shown in the fig. Here, we can observe that the feasible region is bounded.

The coordinates of the corner points A(60,0),B(60,40),C(50,50) and D(10,50).

Now, we find the minimum value of Z. According to table the minimum value of Z = 510 at point D (10,50).
Hence, the amount of grain delivered from A to D, E and F is 10 quintal, 50 quintals and 40 quintals respectively and from B to D,E and F is 50 quintal, 0 quintal and 0 quintal respectively.