A toy company manufactures two types of dolls, A and B. Market tests and available resources have indicated that the combined production level should not exceed 1200 dolls per week and the demand for dolls of type B is at most half of that for dolls of type A. Further, the production level of dolls of type A can exceed three times the production of dolls of other type by at most 600 units. If the company makes profit of Rs 12 and Rs 16 per doll respectively on dolls A and B, how many of each should be produced weekly in order to maximise the profit?
let x and y be the number of dolls of type A and B respectively. Obviously x ≥ 0, y ≥ 0. Mathematical formulation of given problem is as follows:
Maximize Z = 12x + 16y ….(1)
Subject to the constraints,
x + y ≤ 1200 …..(2)
y ≤ x/2 ⇒ 2y ≤ x …..(3)
x – 3y ≤ 600 ….(4)
x ≥ 0, y ≥ 0 ….(5)
Now let us graph the feasible region of the system of inequalities (2) to (5). The feasible region (shaded) is shown in the fig. Here, we can observe that the feasible region is bounded.
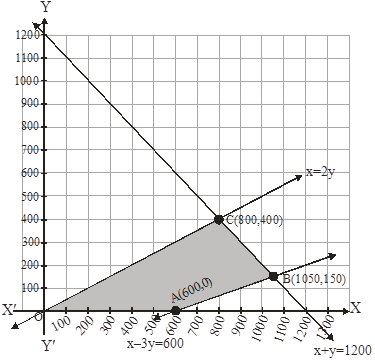
The coordinates of the corner points A(600,0), B(1050,150) and C(800,400).

Now, we find the maximum value of Z. According to table the maximum value of Z = 16000 at point C (800,400).
Hence, 800 and 400 be the number of dolls of type A and B respectively.