The slope of a line is double of the slope of another line. If tangent of the angle between them is 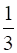 , find the slopes of the lines.
, find the slopes of the lines.
let m1 and m be the slope of the two given lines such that m1= 2m
We know that if θ is the angle between the lines l1 and l2 with slope m1 and m2, then
![]()
Given here that the tangent of the angle between the two lines is ![]()
∴ ![]()
⇒ ![]()
Case 1
⇒ ![]()
⇒ 1+2m2 = -3m
⇒ 2m2 +1 +3m = 0
⇒2m(m+1) + 1(m+1)=0
⇒(2m+1)(m+1)= 0
⇒ m =-1 or ![]()
If m = -1, then the slope of the lines are -1 and -2
If m = ![]() , then the slope of the lines are
, then the slope of the lines are ![]() and -1
and -1
Case 2
⇒![]()
⇒ 2m2 +1 -3m = 0
⇒ m = 1 or 1/2
If m = 1, then the slope of the lines are 1 and 2
If m = ![]() , then the slope of the lines are
, then the slope of the lines are ![]() and 1
and 1
Hence the slope of the lines are -1 and -2 or ![]() and -1 or 1 and 2 or
and -1 or 1 and 2 or ![]() and 1.
and 1.
18