Let AB be the line segment such that R (h, k) divides it in the ratio 1: 2.

Let the coordinates of A and B are (0, y) and (x, 0) respectively.

We know that the coordinates of a point dividing the line segment joining the points (x₁, y₁) and (x₂, y₂) internally in the ratio m: n are .

∴

⇒

∴ h = 2x/3 and k = y/3

⇒ x = 3h/2 and y = k/3

∴ A = (0, k/3) and B = (3h/2, 0)

We know that the equation of the line passing through the points (x₁, y₁) and (x₂, y₂) is given by

∴

⇒

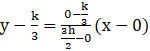
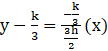
⇒ hy = -2kx + 3hk

∴ 2kx + hy = 3hk

Ans. The equation of line is 2kx + hy = 3hk.