The equations of given lines are

x cos θ – y sin θ = k cos 2θ …………………… (1)

x sec θ + y cosec θ = k ……………….… (2)

Perpendicular distance (d) of a line Ax + By + C = 0 from a point (x₁, y₁) is given by

Comparing equation (1) to the general equation of line i.e., Ax + By + C = 0, we obtain

A = cos θ, B = -sin θ, and C = -k cos 2θ

Given that p is the length of the perpendicular from (0, 0) to line (1).

∴

p = k cos 2θ

Squaring both sides

P² = k² cos²2θ …………………(3)

Comparing equation (2) to the general equation of line i.e., Ax + By + C = 0, we obtain

A = sec θ, B = cosec θ, and C = -k

Given that q is the length of the perpendicular from (0, 0) to line (2)

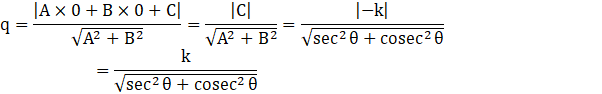
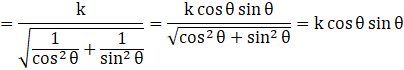
Multiplying both sides by 2

2q = 2k cos θ sin θ = k × 2sinθ cosθ

2q = k sin 2θ

Squaring both sides

4q² = k² sin²2θ …………………(4)

Adding (3) and (4) we get

p² + 4q² = k² cos² 2θ + k² sin² 2θ

⇒ p² + 4q² = k² (cos² 2θ + sin² 2θ) [∵cos² 2θ + sin² 2θ = 1]

∴ p² + 4q² = k²