Find the direction in which a straight line must be drawn through the point (–1, 2) so that its point of intersection with the line x + y = 4 may be at a distance of 3 units from this point.
Let y = mx + c be the line through points (-1, 2).
2 = m (-1) + c
⇒ c = m + 2
Thus, y = mx + m + 2 --------------- (1)
The given line as
x + y = 4 ------------ (2)
On solving these equations, we get,
and 
Thus, 
is the point of intersection of lines (1) and (2).
Since, this point is at a distance of 3 units from point (-1, 2),
So, now using distance formula,
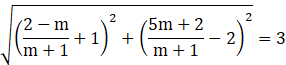
⇒ 1 + m2 = m2 + 1 + 2m
⇒ 2m = 0
⇒ m = 0
Therefore, the slope of the required line must be zero,
Hence, the line must be parallel to the x – axis.
16