The equation of the given lines are

y = 3x +1 ------------ (1)

2y = x + 3 ------------- (2)

y = mx + 4 ------------- (3)

Slope of line (1), m₁ = 3

Slope of line (2), m₂ =

Slope of line (3), m₃ = m

It is given that lines (1) and (2) are equally inclined to line (3).

So, the angle between lines (1) and (3) equals between lines (2) and (3).

Thus,

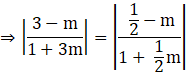
or

Now, if , we get,

(3 – m)(m +2) = (1 – 2m)(1 +3m)

⇒ -m² + m + 6 = 1 + m – 6m²

⇒ 5m² + 5 = 0

⇒ (m + 1) = 0

⇒ m = , which is not real

If , then

(3 – m)(m +2) = - (1 – 2m) (1 +3m)

⇒ -m² + m + 6 = -(1 + m – 6m²)

⇒ 7m² + 2m - 7 = 0

⇒ m =

⇒ m =

⇒ m =

Therefore, the required value of m is.