Given: ABCD is a rhombus

∠ACB = 40°

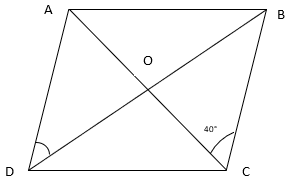
∵ ∠ACB = 40°

⇒ ∠OCB = 40°

∵ AD ∥ BC

⇒ ∠DAC = ∠BCA = 40° [Alternate interior angles]

⇒ ∠DAO = 40°

Since, diagonals of a rhombus are perpendicular to each other

∴ ∠AOD = 90°

Sum of all angles of a triangle is 180°

⇒ ∠AOD + ∠ADO + ∠DAO = 180°

⇒ 90° + ∠ADO + 40° = 180°

⇒ 130° + ∠ADO = 180°

⇒ ∠ADO = 180° – 130°

⇒ ∠ADO = 150°

⇒ ∠ADB = 150°

Hence, ∠ADB = 150°