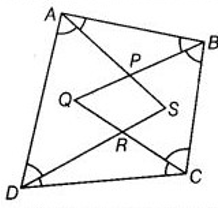
Sum of all angles of a quadrilateral is 360°

⇒ ∠A + ∠B + ∠C + ∠D = 360°

On dividing both sides by 2,

⇒ 1/2(∠A + ∠B + ∠C + ∠D) = 1/2 × 360° = 180°

∵ AP, PB, RC and RD are bisectors of ∠A, ∠B, ∠C and ∠D

⇒ ∠PAB + ∠ABB + ∠RCD + ∠RDC = 180° …(1)

Sum of all angles of a triangle is 180°

∴ ∠PAB + ∠APB + ∠ABP = 180°

⇒ ∠PAB + ∠ABP = 180° – ∠APB …(2)

Similarly,

∴ ∠RDC + ∠RCD + ∠CRD = 180°

⇒ ∠RDC + ∠RCD = 180° – ∠CRD …(3)

Putting (2) and (3) in (1),

180° – ∠APB + 180° – ∠CRD = 180°

⇒ 360° – ∠APB – ∠CRD = 180°

⇒ ∠APB + ∠CRD = 360° – 180°

⇒ ∠APB + ∠CRD = 180° …(4)

Now,

∠SPQ = ∠APB [vertically opposite angles]

∠SRQ = ∠DRC [vertically opposite angles]

Putting in (4),

⇒ ∠SPQ + ∠SRQ = 180°

Hence, PQRS is a quadrilateral whose opposite angles are supplementary.