The Midpoint Theorem states that the segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side.

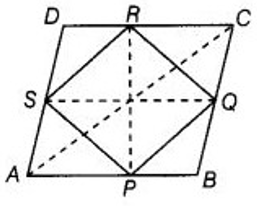
Join AC, RP and SQ

In ∆ABC,

P is midpoint of AB and Q is midpoint of BC

∴ By midpoint theorem,

PQ ∥ AC and PQ = 1/2AC …(1)

Similarly,

In ∆DAC,

S is midpoint of AD and R is midpoint of CD

∴ By midpoint theorem,

SR ∥ AC and SR = 1/2AC …(2)

From (1) and (2),

PQ ∥ SR and PQ = SR

⇒ PQRS is a parallelogram

ABQS is a parallelogram

⇒ AB = SQ

PBCR is a parallelogram

⇒ BC = PR

⇒ AB = PR [∵ BC = AB, sides of rhombus]

⇒ SQ = PR

∴ diagonals of the parallelogram are equal

Hence, it is a rectangle.