The Midpoint Theorem states that the segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side.

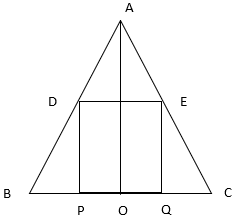
In ∆ABC,

D and E are midpoints of AB and AC

By midpoint theorem,

DE ∥ BC and DE = 1/2BC

⇒ DE = 1/2[BP + PO + OQ + QC]

∵ P and Q are midpoints of OB and OC

⇒ DE = 1/2[2PO + 2OQ]

⇒ DE = PO + OQ

⇒ DE = PQ …(1)

Now,

In ∆AOC,

Q and C are midpoints of OC and AC

By midpoint theorem,

EQ ∥ AO and EQ = 1/2AO …(2)

Similarly,

In ∆AOB,

D and P are midpoints of AB and OB

By midpoint theorem,

PD ∥ AO and PD = 1/2AO …(3)

Also,

By midpoint theorem,

DE ∥ BC and DE = 1/2BC …(4)

From (2) and (3),

EQ ∥ PD and EQ = PD

From (1) and (4),

DE ∥ BC

DE ∥ PQ and DE = PQ

Hence, DEPQ is a parallelogram