D and E are the mid-points of the sides AB and AC respectively of DABC. DE is produced to F. To prove that CF is equal and parallel to DA, we need an additional information which is

Question

Philoid · Accepted Answer

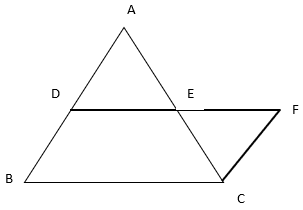
Let us assume that, DE = EF

AE = CE [∵ E is mid-point of AC]

DE = EF [assumed]

∠AED = ∠FEC [vertically opposite angles]

∴ By SAS, ∆AED ≅ ∆FEC

∴ By CPCT, AD = CF and ∠ADE = ∠CFE

∵ alternate interior angles are equal

∴ AD ∥ CF

That proves our assumption was correct

Hence, DE = EF