P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
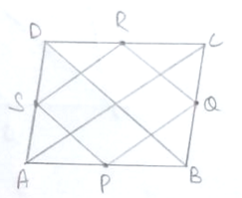
Given, P, Q, R and S are mid-points of the sides AB, BC, CD and DA, respectively.
Also, AC = BD.
In ΔADC, by mid-point theorem,
SR||AC and SR = 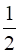 AC
AC
In ΔABC, by mid-point theorem,
PQ||AC and PQ = 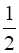 AC
AC
![]() SR = PQ =
SR = PQ = 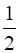 AC
AC
Similarly,
In ΔBCD, by mid-point theorem,
RQ||BD and RQ = 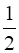 BD
BD
In ΔBAD, by mid-point theorem,
SP||BD and SP =  BD
BD
![]() SP = RQ =
SP = RQ = 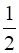 BD =
BD =  AC
AC
So,
SR = PQ = SP = RQ
Hence, PQRS is a rhombus.
4