Given, ABCD is a trapezium in which AB||CD. Also, E and F are mid-points of sides AD and BC.

Now, joining BE and produce it to meet CD produced at G.

In ΔGCB, by mid-point theorem

EF||GC

But GC = CD, and CD||AB

EF||AB

Now, draw BD which intersects EF at O.

In ΔADB, AB||EO and E is mid-point of AD.

By converse of mid-point theorem, O is mid-point of BD.

Also, EO = 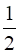AB …(i)

In ΔBDC, OF||CD and O is mid-point of BD.

OF = 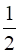CD …(ii)

Adding (i) and (ii),

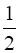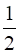EO + OF = AB + CD

EF = 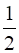(AB + CD)

Hence, proved.