In Fig.10.15, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD + ∠BED.

Question

In Fig.10.15, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ ACD + ∠ BED.

Philoid · Accepted Answer

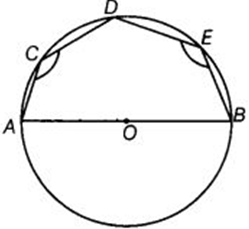
Given: AOB is the diameter.

Join AE and ACDE is a cyclic quadrilateral.

∴ ∠ACD + ∠AED = 180° (sum of opposite angles of a cyclic quadrilateral is 180°) ………………(1)

As diameter subtend a right angle to the circle, therefore,

∠AEB = 90° ………………(2)

Adding equation (1) and (2), we have:

∠ACD + ∠AED + ∠AEB = 180° + 90°

⇒ ∠ACD + ∠BED = 270°