Let the given right angled triangle be ΔABC.

Steps of Construction:

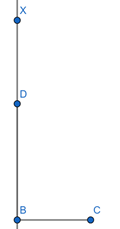
(i) Draw base, BC = 3.5 cm

Now, draw a ray BX such that ∠XBC = ∠B = 90°.

(ii) Here, sum of two sides = AB + AC = 5.5 cm

So, cut the line segment BD = 5.5 cm from ray BX.

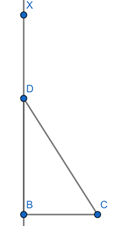
(iii) Join DC.

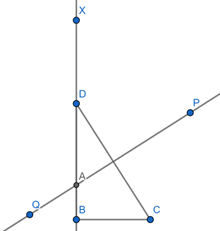
(iv)Draw perpendicular bisector of DC, say PQ, which intersects BD at A.

(v) Now join AC.

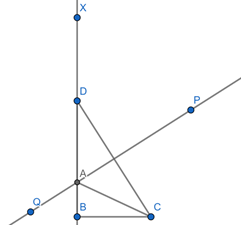
Thus, we get a ΔABC which is the required triangle.

Justification:

Base BC and ∠B are drawn as given.

Since, PQ is perpendicular bisector of CD and A lies on it.

∴ AD = AC

Now, AB = BD – AD

= BD – AC

∴ AB + AC = BD

Thus, construction is justified.