If 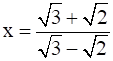 and
and 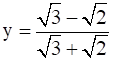 , then find the value of x2 + y2.
, then find the value of x2 + y2.
![]()
(a + b) 2 = a2 + 2ab + b2
Also x = 1 / y or y = 1/x
Let a = x
b = y
(x + y) 2 = x2 + 2xy + y2
But we know y = 1/x
![]()
![]()
![]()
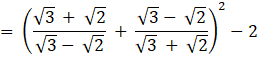
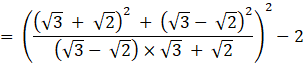
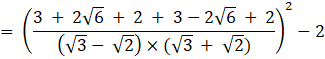
Here the denominators form the expansion as
(a + b) × (a – b) = (a2 – b2)
Here a = √3
b = √2
a2 = (√3)2
= 3
b2 = (√2)2
= 2
![]()
= 102 – 2
= 100-2
= 98
5