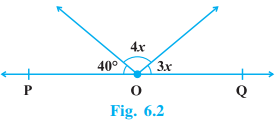
It is given to us that POQ is a line.

From the figure, we can say that OA and OB are two lines intersecting on the line POQ.

We know,

If a ray stands on a line, then the sum of the two adjacent angles so formed is 180°. - - - - (i)

So, ∠POA + ∠AOB + ∠BOQ = 180°

⇒ 40° + 4x + 3x = 180° (Given - ∠POA = 40°, ∠AOB = 4x, ∠BOQ = 3x)

⇒ 7x = 140°

⇒ x = 20°

Thus, option (A) is the right answer.

Option (B) is not correct because on putting x = 25° on ∠AOB = 4x and on ∠BOQ = 3x, the theorem (i) is not satisfied, i.e.,

∠POA + ∠AOB + ∠BOQ ≠180°

Thus, the value of x is not 25°.

Option (C) is not correct because on putting x = 30° on ∠AOB = 4x and on ∠BOQ = 3x, the theorem (i) is not satisfied, i.e.,

∠POA + ∠AOB + ∠BOQ ≠180°

Thus, the value of x is not 30°.

Option (D) is not correct because on putting x = 25° on ∠AOB = 4x and on ∠BOQ = 3x, the theorem (i) is not satisfied, i.e.,

∠POA + ∠AOB + ∠BOQ ≠180°

Thus, the value of x is not 25°.