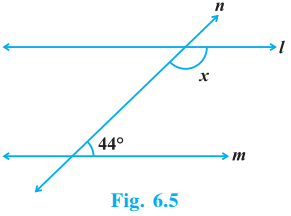
In Fig. 6.5, find the value of x for which the lines l and m are parallel.
Let us draw the figure as below -
It is given to us that l and m are parallel to each other.
Here, n is a transversal intersecting l and m which are parallel to each other.
Also, we have ∠pqm = 44° - - - - (i)
We have to find the value of x, i.e., ∠qpl
We know, if a transversal intersects two parallel lines then each pair of corresponding angles is equal.
Here, the transversal n intersects two parallel lines l and m. So, the following holds true for the corresponding angles.
∠pqm = ∠npl
⇒ ∠npl = 44° (From (i), we have ∠pqm = 44°) - - - - (ii)
Again, the linear pair axiom states that
If a ray stands on a line, then the sum of two adjacent angles so formed is 180°.
Here, we can see that l is a ray standing on the line n.
⇒ ∠npl + ∠lpq = 180° (By linear pair axiom)
⇒ 44° + ∠lpq = 180°
⇒ ∠lpq = 180° - 44°
⇒ ∠lpq = 136°
⇒ x = 136°
Thus, the value of x is equal to 136°.