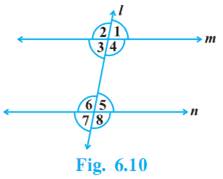
It is given to us –

∠1 = 60°

∠6 = 120°

We have to show that m and n are parallel to each other.

We can see that l is a ray standing on the line m. So, by linear pair axiom,

∠1 + ∠4 = 180°

⇒ 60° + ∠4 = 180°

⇒ ∠4 = 180° - 60°

⇒ ∠4 = 120° - - - - (i)

Similarly,

∠1 + ∠2 = 180°

⇒ 60° + ∠2 = 180°

⇒ ∠2 = 180° - 60°

⇒ ∠2 = 120° - - - - (ii)

Again, ∠2 + ∠3 = 180°

⇒ 120° + ∠3 = 180°

⇒ ∠3 = 180° - 120°

⇒ ∠3 = 60° - - - - (iii)

Since, ∠6 = 120° and ∠2 = 120° [from equation (ii)],

We can say that these corresponding angles are equal, i.e.,

∠6 = ∠2 = 120° - - - - (iv)

We can say that l is a ray standing on the line n. By linear pair axiom,

∠6 + ∠5 = 180°

⇒ 120° + ∠5 = 180°

⇒ ∠5 = 180° - 120°

⇒ ∠5 = 60° - - - - (v)

Since, ∠1 = 60° and ∠5 = 60° [from equation (v)],

We can say that these corresponding angles are equal, i.e.,

∠1 = ∠5 = 60° - - - - (vi)

Similarly, we get

∠8 = ∠4 = 120° (which are also the corresponding angles, and from equation (i), ∠4 = 120°)

And, ∠7 = ∠3 = 60° (which are also the corresponding angles, and from equation (iii), we have ∠3 = 60°)

Thus, we can say that

l is a transversal intersecting two lines m and n such that each pair of corresponding angles are equal.

Then, lines m and n are parallel to each other.