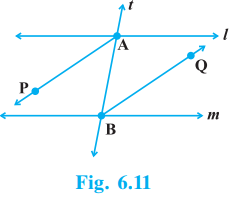
It is given to us –

l and m are two parallel lines.

t is a transversal intersecting l and m at A and B respectively.

Here, ∠EAB and ∠ABH, ∠FAB and ∠GBA, are a pair of alternate interior angles which are equal to each other.

⇒ ∠EAB = ∠ABH, and ∠FAB = ∠GBA - - - - (i)

AP and BQ are the bisectors of the angles ∠EAB and ∠ABH respectively.

⇒ ∠EAP = ∠PAB, and ∠ABQ = ∠QBH - - - - (ii)

From equation (i), we have

∠EAB = ∠ABH

⇒ ∠EAP + ∠PAB = ∠ABQ + ∠QBH (From the figure)

⇒ 2 × ∠PAB = 2 × ∠ABQ [From equation (ii)]

⇒ ∠PAB = ∠ABQ - - - - (iii)

Now, t is a transversal intersecting two lines AP and BQ at A and B respectively.

⇒ ∠PAB and ∠ABQ are a pair of alternate interior angles.

Thus, from equation (iii) we can say that ∠PAB and ∠ABQ are a pair of alternate interior angles, which are equal to each other.

Thus, it is true that AP || BQ.